Is it possible to measure all possible integer lengths on a ruler without marking every integer on that ruler? This is an engaging and challenging problem for all. Beautiful mathematics can be revealed while delving deeper into this rich mathematical task.

A decade ago, while researching the expected value for lottery tickets in various states, a group of MIT students won millions of dollars in the Massachusetts $2 Cash Winfall drawing. Do you want to know how they did it? This teacher led activity starts with a lottery, explores expected value, and finally ties into finite projective geometries.

Many topics in mathematics can be made much clearer when symmetric aspects are made clear or when nice alternative visualizations are possible. When this occurs, it helps both the student and the teacher. We will examine visualization and symmetry in a very general way by means of a set of problems.

Developed as part of the Math Circles of Inquiry project, this five to six day activity is designed to help students understand trigonometric ratios, by building on their understanding of similar triangles and ratios of corresponding sides.

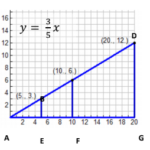
We adapt “Parable of the Polygons” (Vi Hart and Nicky Case), an online simulation on diversity and segregation, into an appropriate MTC session. The session is interactive, and offers multiple layers of content depending on the age and comfort level of students with conversations on social issues.

Are there more fractions than counting numbers? Surprisingly, an investigation into binary notation can help us answer this question! This session explores the binary number system. Participants will investigate Hyperbinary numbers, create a Fraction Tree, and discover connections between them.

A pentagonal tiling is a tiling of the plane where each individual piece is in the shape of a pentagon. The plane cannot be tiled with regular pentagons. However, are there any convex pentagons that can tile the plane? This session explores various pentagons and their tiling abilities.

The game of Tic-Tac-Toe has roots going back centuries. Grid-style game boards have been found in Ancient Egypt, during the Roman Empire, and in our current age on restaurant placemats. Multiple avenues of exploration are possible with this simple children's game. A related game called “Gobblet Gobblers” takes Tic-Tac-Toe to a whole new level!

College students need to be matched with a roommate. They each make a list of who they prefer to room with. Given the preference lists for each individual, can we find a matching that is stable? That is, would any pair ask to change rooms because they would rather room together than with their current roommates? Explorations lead to new questions or new avenues to investigate using various mathematical methods including, but not limited to, combinatorics, graph theory, or matrices.

A Mad Veterinarian has created three animal transmogrifying machines… While grappling with the posed questions, players will explore a set of problems, figuring out how and if the machines can complete a given transformation. Connections can be made to invariants, abstract algebra, graph theory, and Leavitt path algebra.